Research
I am a postdoc in applied analysis with research ambitions located at the intersection of mean-field particle models, network dynamics, mathematics of machine learning and control theory.
Currently, I am work on my own project at TU Vienna in the Institut of Analysis and Scientific Computing in the research group of Ansgar Jüngel.
Before that:- Post-doc at Department of Mathematics, TU Munich, Research Group on Dynamical Systems with Christian Kuehn via Schrödinger program (Austiran Science Fund).
- Post-doc at Department of Data Science, FAU Erlangen, Group of Enrique Zuazua.
- PhD from the TU Vienna, Group of Anton Arnold (2020).
- Invited guest of Shi Jin at Shanghai Jiao Tong University (2019).
Large-time behavior of mean-field equations
My PDE analysis focuses on obtaining explicit large-time estimates for mean-field equations by means of entropy methods and spectral theory. I am specifically interested in including long-range particle interactions on large networks in these models, which have many fascinating real-world applications, such as synchronisation phenomena, opinion formations and neural network dynamics.
Mathematical foundation of machine learning
The achievements of machine learning algorithms backed by huge computing resources are reshaping our lives, but still surprisingly little is understood about the fundamental mechanisms involved. As a mathematician, my goal is to look inside the black box and develop a vocabulary to describe the observed dynamics which at this point seem like magic. I am currently investigating deep learning algorithms and their lack of robustness from an optimal control and dynamical system perspective.

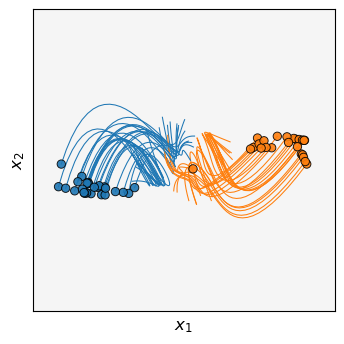
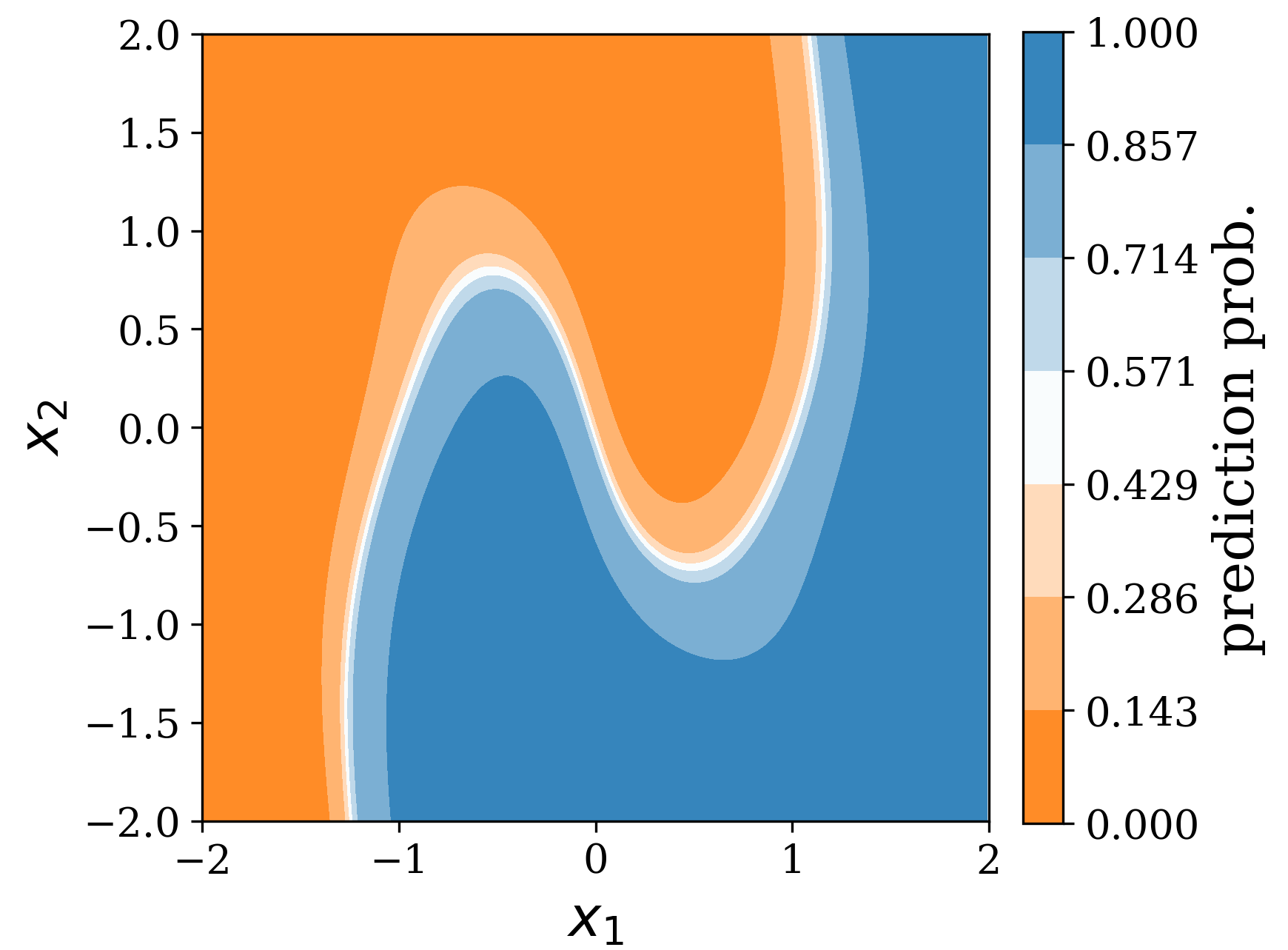
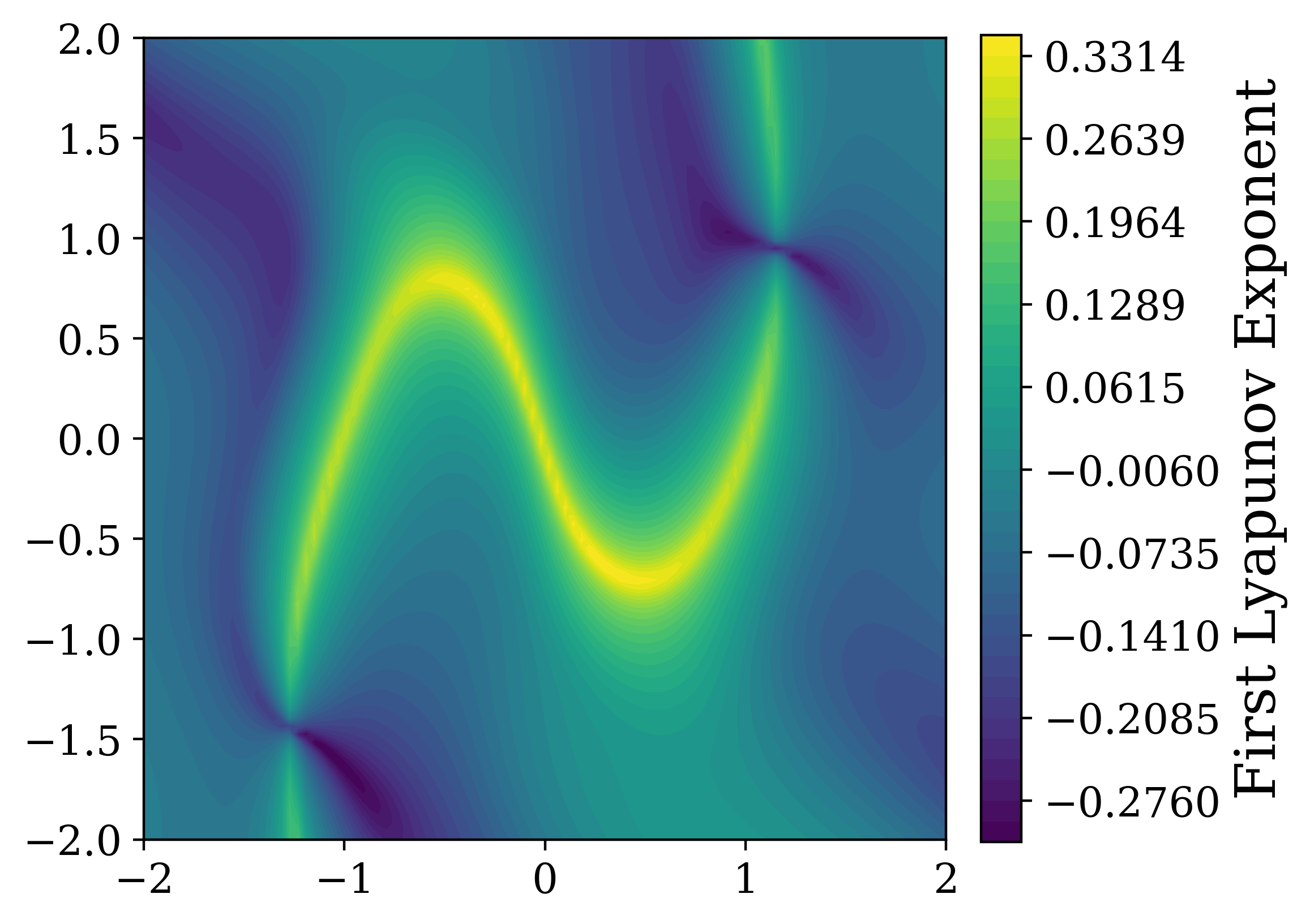
Publications
- Global stability for McKean-Vlasov equations on large networks, Kuehn, C. and Wöhrer, T; European Journal of Applied Mathematics (2024).
- A minimax optimal control approach for robust neural ODEs, Cipriani, C., Scagliotti, A. and Wöhrer, T; European Control Conference (2024).
- Sharp Decay of the Fisher Information for Degenerate Fokker-Planck Equations, Arnold, A., Einav, A. and Wöhrer, T.; Submitted (2023).
- Generalised Fisher Information Approach to Defective Fokker-Planck Equations, Arnold, A., Einav, A. and Wöhrer, T.; Submitted (2022).
- Sharpening of decay rates in Fourier based hypocoercivity methods, Arnold, A., Dolbeault, J., Schmeiser, C. and Wöhrer, T.; Springer INdAM Series: Recent Advances in Kinetic Equations and Application, 48, (2021).
- Large time convergence of the non-homogeneous Goldstein–Taylor Equation, Arnold, A., Einav, A., Signorello B. and Wöhrer, T.; Journal of Statistical Physics, 182 (41) (2021).
- Sharp decay estimates in local sensitivity analysis for evolution equations with uncertainties: from ODEs to linear kinetic equations, Arnold, A., Jin, S. and Wöhrer, T.; Journal of Differential Equations, 268 (3), 1156–1204,(2020).
- On the rates of decay to equilibrium in degenerate and defective Fokker–Planck equations, Arnold, A., Einav, A. and Wöhrer, T.; Journal of Differential Equations, 264 (11), 6843–6872, (2018).
- Asymptotic behavior of the Weyl m-function for one-dimensional Schrödinger operators with measure-valued potentials , Luger, A., Teschl, G. and Wöhrer, T.; Monatsh. Math. 179, 603–613, (2016).
"The writers who embellish a language, who treat it as an object of art, make of it at the same time a more supple instrument, more apt for rendering shades of thought."
Henri Poincaré.
In my sparetime I enjoy exploring the world through photography.


